1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
58
59
60
61
62
63
64
65
66
67
68
69
70
71
72
73
74
75
76
77
78
79
80
81
82
83
84
85
86
87
88
89
90
91
92
93
94
95
96
97
98
99
100
101
102
103
104
105
106
107
108
109
110
111
112
113
114
115
116
117
118
119
120
121
122
123
124
125
126
127
128
129
130
131
132
133
134
135
136
137
138
139
140
141
142
143
144
145
146
147
148
149
150
151
152
153
154
155
156
157
158
159
160
161
162
163
164
165
166
167
168
169
170
171
172
173
174
175
176
177
178
179
180
181
182
183
184
185
186
187
188 
e weights for the practical mat
Page 48
Page 49
If you've noticed an error in this article please click here to report it so we can fix it.
The CPC exam question on calculating axle weights often has practical men flummoxed, Here Les Oldridge Makes things clec
THE SYLLABUS of the Royal Society of Arts examination for the Certificate of Professional Competence in Road Transport. contains an. item (B1. 2:3): "State methods of calculating axle weights and weight distribution".
To practical men, who left school a long time ago, this item is difficult and a simple explanation is hard to find. The subject which deals with problems of this kind is "moments", the moment of a force being defined as the turning effect of a. force.
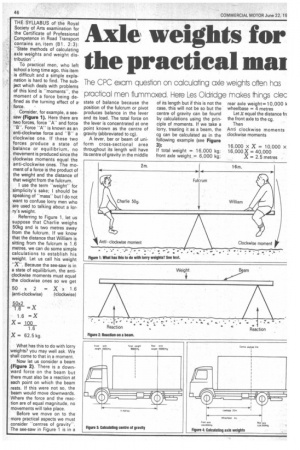
Consider, for example, a seesaw (Figure 1). Here there are two forces, force "A" and force "B". Force "A" is known as an anti-clockwise force and "B" a clockwise one. If these two forces produce a state of balance or equilibrium, no movement is produced since the clockwise moments equal the anti-clockwise ones. The moment of a force is the product of the weight and the distance of that weight from the fulcrum.
I use the term "weight" for simplicity's sake; I should be speaking of "mass" but I do not want to confuse lorry men who are used to talking about a lorry's weight.
Referring to Figure 1, let us suppose that Charlie weighs 50kg and is two metres away from the fulcrum. If we know that the distance that William is sitting from the fulcrum is 1.6 metres, we can do some simple calculations to establish his weight. Let us call his weight "X". Because the see-saw is in a state of equilibrium, the anticlockwise moments must equal the clockwise ones so we get
50 x 2 = x 1.6
(anti-clockwise) ('clockwise) 50x2
1.6 = X 1.6 =X
X= 100 1.6 X= 62.5 kg.
What has this to do with lorry weights? you may well ask. We shall come to that in a moment.
Now let us consider a beam (Figure 2). There is a downward force on the beam but there must also be a reaction at each point on which the beam rests. If this were not so, the beam would move downwards. Where the force and the reaction are of equal magnitude, no movements will take place.
Before we move on to the more practical aspects we must consider "centres of gravity". The see-saw in Figure 1 is in a state of balance because the position of the fulcrum or pivot produces balance in the lever and its load. The total force on the lever is concentrated at one point known as the centre of gravity (abbreviated to cg).
A lever, bar or beam of uniform cross-sectional area throughout its length will have its centre of gravity in the middle of its length but if this is not the case, this will not be so but the centre of gravity can be found by calculations using the principle of moments. If we take a lorry, treating it as a beam, the cg can be calculated as in the following example (see Figure 3): If total weight = 16,000 kg; front axle weight = 6,000 kg; rear axle weight = 10,000 k wheelbase = 4 metres Let X equal the distance frc the front axle to the cg.
Then Anti clockwise moments clockwise moments
16,000 x X = 10,000 x
16,000X = 40,000 X = 2.5 metres his is still not what we are ing for; it is not what the man requires because he Js to know axle weights, but are getting near to our ictive now. Taking another • at our last calculation
ii weight X distance from Yt axle to cg = rear axle ght x wheelbase
I weight X distance from it axle to cg wheelbase ear axle weight
low we can look at the pracaspects. Instead of using term centre of gravity, let us ;ider the centre payload line I uniformly loaded vehicle. lure 4). Using the centre of front wheel as the datum, distance to the payload line _) and the distance between axles can be measured. The ler is the loadbase and the 3r the wheelbase. The foling formula, derived from one above will now form the
s of all load calculations: 'ayload on rear axle = payload X loadbase wheelbase
-he following example shows if the formula works.
inladen axle weights front 2000 kg Rea 'r 3000 kg load = 9000 kg dbase = 2.5 metres eelbase = 4 metres aunt of payload on rear axle = Payload X Loadbase Wheelbase = 9000 X 2.5 4 = 22,500 4 = 5625 kg (punt of payload on front axle ayload — rear axle payload 3000 — 5625 3375 kg
al weight on rear axle )ayload on rear axle JW on rear axle
5625 + 3000 3625 kg al weight on front axle
JW on front axle + Payload Dn front axle
2000 + 3375 5375 kg For examination purposes all t is really necessary is to morise the last formula I have en and then be able to calcu: front and rear axle weights
Ti it.