EQUIPPING A COACH FOR WIRELESS RECEPTION.
Page 24
If you've noticed an error in this article please click here to report it so we can fix it.
The Exact Nature of the Phenomenon of Resonance whereby the Tuning of Radio Circuits Becomes Possible is Further Dealt With in this Article.
WE SAW, in the 'last article dealing with the interesting phenomena of wireless reception, that resonance, which is a property cominon to all motion of an alternating or oscillatory nature, could be illustrated by a few simple experiments on such a common, or garden, thing as a child's swing. We) moreover, learnt that the case of the garden swing could be exactly duplicated by that of a tightly stretched string, such as is used in a great number of musical instruments. We will now detail one more experiment with the string which will prove that it also forms a true resonant system.
In the case of the swing, as is pretty common knowledge, we stated that the pushes or impulses given to it Must be tithed exactly to coincide with the time period of the swing, otherwise the swing, instead of speeding up, would slow down. And, in the castof the string, we may expect that the same thing will take place. The child's swing, of course, oscillates quite slowly, and so it is easy to time our pushes to its natural period, but the string vibrates at a very much greater speed, hence the matter is not quite so simple in that case.
Now, when we wish to set a stretched string in motion we can either pluck it, or blow it, or hit it. In each case we give it a shock and,.after this initial excitation, we leave it alone to vibrate at its own resonant frequency. This is tantamount to giving the swing one hefty push and then walking away and leaving it. Such shock excitation takes place in radio when we receive signals from spark transmitters and also when we get atmospherics—those unwelcome interferers from " Nature's wireless stationf.' If, however, we want to set the string in motion other than by an initial shock we muse-find some means of giving it a series oflittle pushes in time with its own frequency. Luckily, in our own throats we have the apparatus at hand. If we have a string stretched until it. sounds a certain musical note, and if we sing close to it that same note, we shall find that the string will respond. The sound waves leasing us—consisting, as they do, of a number of compressions and rarefactions of the
E38
atmosphere, the frequency of which correspond (provided we sing the same. nate). to the period of the string—act on the. latter in just the same way as the small pushes on our swing, and, individually feeble as they are, gradually, owing to resonance, set the string in motion just as effectively as if we had plucked or hit it.
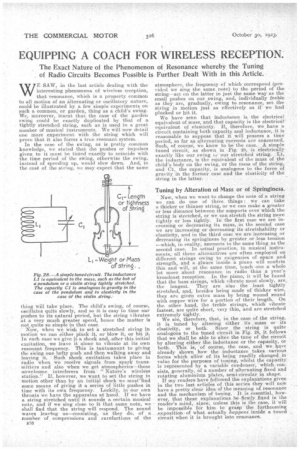
We have seen that inductance is the electrical equivalent of mass, and that capacity is the electrical' equivalent of elasticity. If therefore, we_havc a circuit containing both capacity and. inductance, it is reasonable to suppose that it will possess a time period,.so far as alternating Currents are concerned. Such, of course', We know to be the case. A simple tuned circuit, as shown in Pig. 28, is electrically exactly like our swing GC our stretched string. Li, the inductance, is the equivalent of the mass of the child's body on the swing, of the Mass of the string, and 01, the caitheity, is analogous to the force of gravity in the former case and the elasticity of the string in the latter.
Tuning by Alteration of Mass or of Springiness.
Now, when we want to change the note of a string we can do one of three. things : we can take a thicker or thinner string, or we can make a greater or less distance between the supports over which the' string is stretched, or We can stretch the string More -tightly or less tightly. Inthe first case we are increasing or decreasing its mass, in the second case we are increasing or decreasing its stretchability or elasticity, and in the third case we are increasing or .decreasing its springiness by greater or less tension —which, in reality, amounts to the same thing as the second case. In actual practice, in musical instruments,all three alternatives are often employed on different strings owing to exigencies of space and strength, and a glance inside a piano will confirm this and will, at the same time, teach one a whole lot more about resonance in radio than a year's broadcast reception. In the piano, it will be found that the bass strings, which vibrate most slowly, are, the longest. They are also the least tightly stretched, and, besides being made of thicker wire, they are given extra mass by being wound round with copper wire for a portion of their length. On the other hand, the treble strings, which vibrate fastest, are quite short, very thin, and are stretched extremely tightly.
We see, therefore, that, in the case of the string, it is tuned by altering either its mass or its elasticity, or both. Since the string is quite analogous to the tuned circuit in Fig. 28, it follows that we shall be able to alter the tune of the circuit by altering either, the inductance or the capacity, or both. This is, of course, the case, and we have already shown how the inductance takes .various forms which allhw of its being readily changed in value for the purposes of tuning, whilst the capaeity is represented by a variable condenser, which consists, generally, of a number of alternating fixed and rotating aluminium plates, semi-circular in shape.
If my readers have followed the explanations given in the two last articles of this series they will now have a pretty dear idea of the meaning of resonance and the mechanism of tuning. It is essential, however, that these explanations be firmly, fixed in the reader's mind, since, unless this is the case, it'will be impossible for him to grasp the, forthcoming exposition of what actually happens inside a tuned circuit when it is brought into resonance.




































